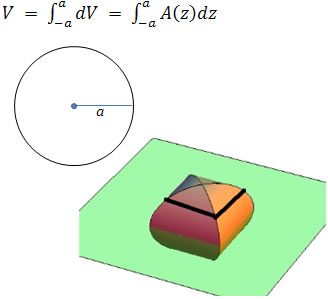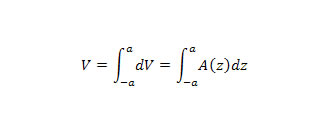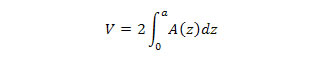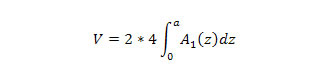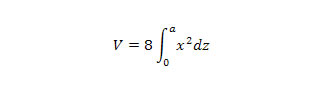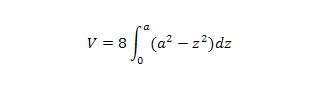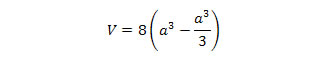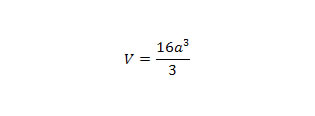Steinmetz Intersection of Cylinders—The Perpendicular Intersection of Two Identical Cylinders

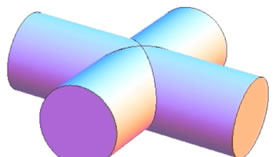
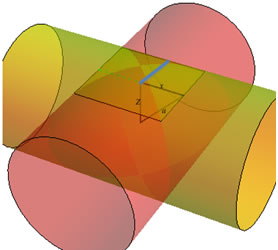
This lesson is about finding the volume of the intersection of two cylinders that are the same size (radius) and intersect at right angles. This is sometimes called a Steinmetz intersection because Charles Steinmetz worked out many arbitrary cases of cylinder intersection.

The problem was introduced when some engineers at General Electric came to Steinmetz around the turn of the twentieth century and asked him if he could find the volume of the intersection of two cylinders coming together at right angles.

Why would we want to know that?

This problem is useful because it has many applications. One such application is demonstrated at Metro Center in Washington, D.C., where two cylindrical subway tunnels intersect in the main area.

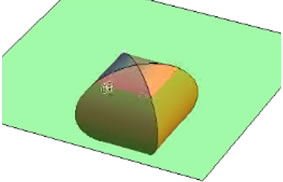
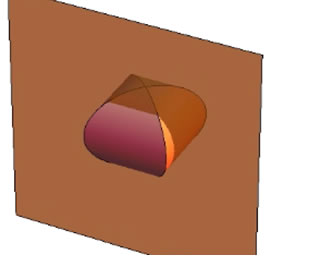
Here is a visual representation, using Mathematica, of the intersection.

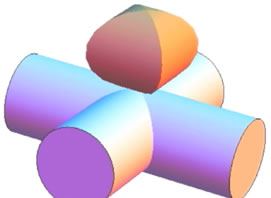
In Mathematica, the intersection volume can be "lifted" out so that we can visualize it.

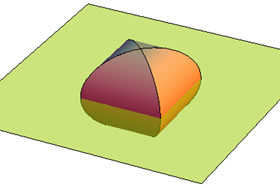
Slicing this volume of intersection with a plane perpendicular to the vertical (z) axis and through the middle (that is, the origin) shows the symmetry between the top and bottom halves. It also indicates that the region of intersection is a square.

Looking back at the intersection of the tunnels at Metro Center, where we are inside the intersection volume, also demonstrates this square appearance.

Here is the same view (inside the cylinder intersection at Metro Center), but with the square intersection highlighted. Note that the figure does not look exactly square due to the perspective of the tunnel.

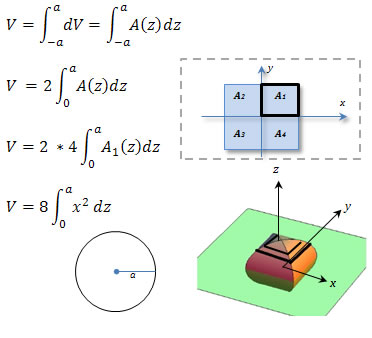
As with the disk method, where a volume is determined by "stacking" disks to fill a region, here we want to slice orthogonal (perpendicular) to the z-axis and "stack" the differential volumes (dV) of these intersections to determine the volume of the cylinder's intersection.

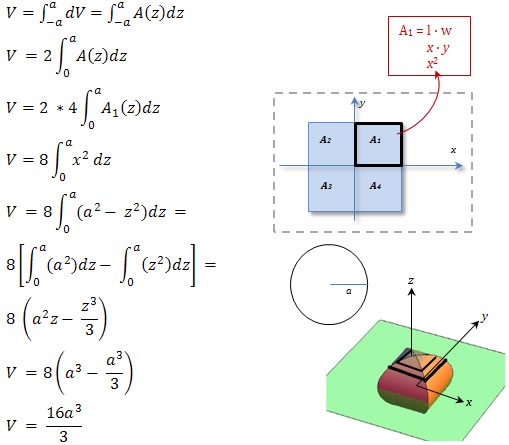
Thus, the volume of intersection is the "sum" (integral) of these differential volumes (dV) from the bottom, where z = −a, to the top, where z = a, where a is the radius of the cylinder.

Furthermore, each differential volume dV is found as the "thickness" dz times the area A(z) of the cross section.

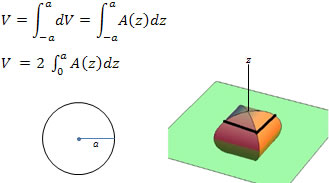
By using the symmetry of the cylinder from bottom to top, the integration can be done from z = 0 to z = a and then doubling the resulting volume.

Thus the total volume is twice the volume of the top half of the intersection volume. To find this, we need to determine the cross-section area, a(z).

Moving the plane of intersection up and down, the z-axis shows that the cross section is, as we demonstrated above, a square. This is because the plane intersects each cylinder along its length, which is a straight line (segment).

Why not take the intersection across the x or y axes? This is certainly possible mathematically, but the region of intersection is not as easily calculated. This is because the intersection is straight along one cylinder, but has curved sides where it intersects the other cylinder along its circular region.

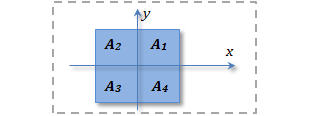
Furthermore, the symmetry of the square can be used to calculate its area, using the area of four smaller, identical areas in each quadrant of the x‑y plane. Notice that the z-axis is at the origin coming straight out (perpendicular) to the page.

By using symmetry, the integral calculation has been reduced to finding the area of the smaller square A₁ that lies in the first quadrant, where all the values will be positive.

So the volume of the intersection will be 8 times the integral from z = 0 to z = a for the differential volume in the first quadrant given by the area of the square.

Thus, we need to determine the area of the square of the intersection in the first quadrant. This square has dimensions x by y, but because it is a square, x and y have the same length, so that the area is just

A₁ = x².

Note that we could just as easily have used y² here.

So the integral is now given by the equation on the left. Note that the integration is with respect to z, but the integrand is a function of x! Furthermore, x is varying (with z), so it cannot be taken outside the integral.

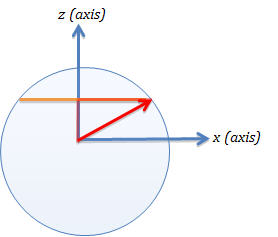
To calculate the integral, the functional relationship between x² and z must be determined. That is, x² needs to be written in terms of z.

Looking at that cylinder intersection above, we see that a right triangle is formed by the z (vertical blue) axis, the x (horizontal blue) axis, and the (red) radius of the cylinder.

The radius (of length a) is the hypotenuse of the right triangle, thus,

x² + z² = a²

or

x² = a² − z²

So, the integral can now be written in terms of z and the antiderivatives found and evaluated. The antiderivative of a² dz is a²z, and that of z² dz is z³.

The evaluation of the antiderivatives at the (lower limit), z = 0, is zero, so only the evaluation at the upper limit, z = a, will determine the volume.

Evaluating and simplifying determines that the volume of the intersection of the cylinders is 16 times the radius of the cylinders cubed, divided by 3.

It is said that Steinmetz did this calculation in his head in less than a minute!