Population Growth
Exercises
Exercise 1
Suppose an alien population, called the Duemans, lives on the second planet around the binary system of Alpha Centauri. Scientists have detected radio signals that indicate the following information about the Dueman population, given in equivalent Earth time:
| Year | Population (in hundred thousands) |
|---|---|
| 1946 | 650 |
| 1950 | 720 |
| 1955 | 810 |
| 1960 | 920 |
| 1965 | 1050 |
| 1970 | 1180 |
| 1975 | 1350 |
| 1980 | 1520 |
| 1985 | 1730 |
| 1990 | 1950 |
| 1995 | 2200 |
| 2000 | 2500 |
| 2005 | 2850 |
| 2010 | 3200 |
Assuming an exponential growth model, determine the growth constant and estimate the Dueman population in the Earth year 2050.
Solution
Exercise 2
Verify that
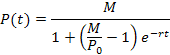
is a solution of the (differential) equation
![]() .
.
Determine the limit of the solution as time t goes to infinity (for r > 0). What is the limit if r > 0 and P0 > M?
Exercise 3
The half-life of decaying radioactive material is analogous to the doubling time of exponential growth. Assuming that a radioactive material takes 12 years to decay to 90 percent of its original weight, what is the half-life of the material?